Elastodynamic guided waves in structures and tissues

Abstract

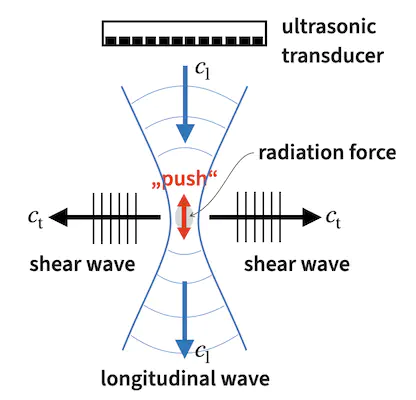
Guided elastic waves emerge in thin or slender structures. They are important for various applications, including evaluation of complex media, sensing, telecommunication, and specific medical diagnosis (elastography). My presentation focuses on the physical peculiarities of guided waves and their manifestation in soft matter akin to biological tissue. The first part of my talk addresses so-called zero-group-velocity (ZGV) resonances, which are guided waves with vanishing power flux. In anisotropic plates, these ZGV resonances induce transverse-group-velocity (TGV) waves, whose power flux is orthogonal to the wave vector. These phenomena can be used effectively for material evaluation. The second part is set in the context of classical shear-wave elastography, which uses shear bulk waves to quantitatively assess the stiffness of large soft tissues. However, its applicability is limited by wave guiding, viscous losses, and pre-stress. I will discuss how to account for these intricate effects to accurately describe guided wave propagation in such media, representing an initial step in broadening the applicability of quantitative elastography.

Daniel A. Kiefer

Researcher at Institut Langevin

Research in guided elastodynamic waves, fluid-structure interaction, simulation and signal processing for ultrasonic sensors and nondestructive testing.